Complex Number Rectangular Form
Complex Number Rectangular Form - Rectangular form is where a complex number is denoted by its respective horizontal and vertical components. #3*cos(120^@)+3*isin(120^@)# recall the unit circle coordinates: Kelley's math & stats help. There's also a graph which shows you the meaning of what you've found. Drive 41 miles west, then turn and drive 18 miles south. The number's \blued {\text {real}} real part and the number's \greend {\text {imaginary}} imaginary part multiplied by i i. Web learn how to convert a complex number from rectangular form to polar form. Find quotients of complex numbers in polar form. Here are some examples of complex numbers in rectangular form. Web the form of the complex number in section 1.1:
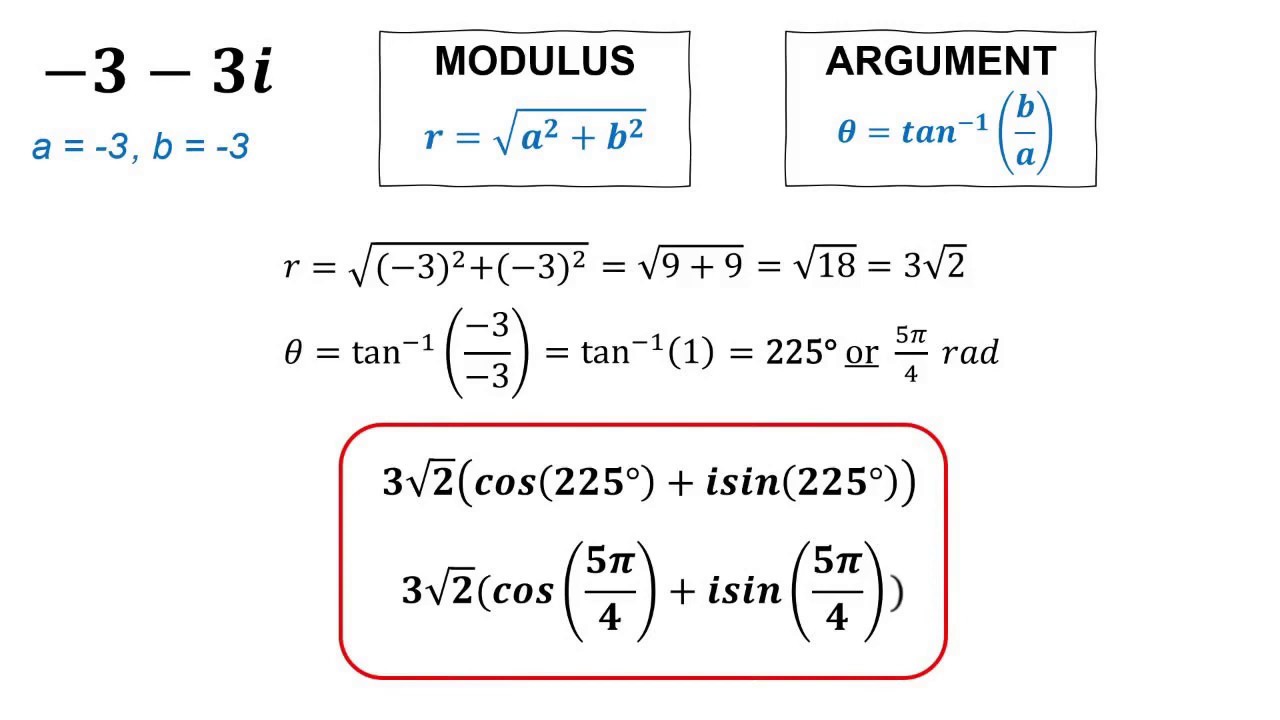
Web polar notation denotes a complex number in terms of its vector’s length and angular direction from the starting point. For example, 2 + 3i is a complex number. Fly 45 miles ∠ 203 o (west by southwest). 5\sqrt {2}\left ( \cos (135\degree) +i\sin (135\degree) \right) 5 2 (cos(135°) +isin(135°)) a 5\sqrt {2}\left ( \cos (135\degree) +i\sin (135\degree) \right) 5 2 The polar form of a complex number z = a + b i is z = r ( cos θ + i sin θ) , where r = | z | = a 2 + b 2 , a = r cos θ and b = r sin θ , and θ = tan − 1 ( b a) for a > 0 and θ = tan − 1 ( b a) + π or θ = tan − 1 ( b a) + 180 ° for a < 0. Find roots of complex numbers in polar form. Web given a complex number in polar form, we can convert that number to rectangular form and plot it on the complex plane. Web what is rectangular form? Z = r(cos(θ) + i ⋅ sin(θ)) we find that the value of r = 4 and the value of θ = π 4. Web learn how to convert a complex number from rectangular form to polar form.
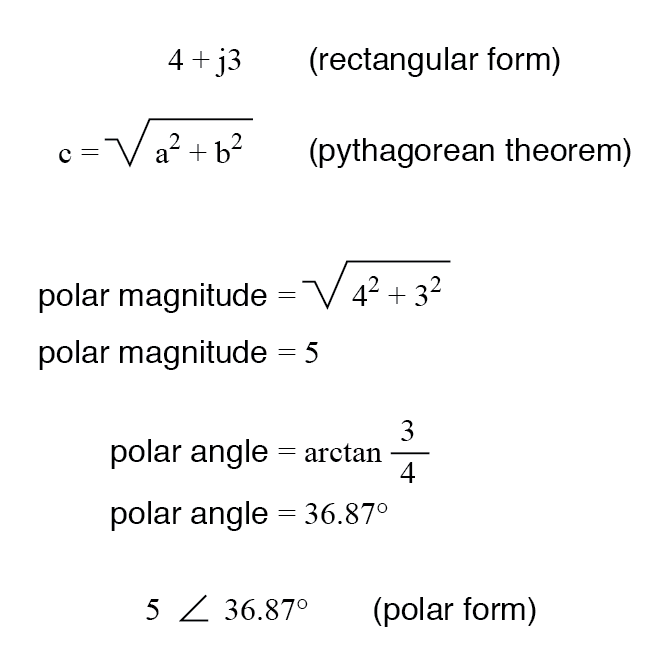
In essence, the angled vector is taken to be the hypotenuse of a right triangle, described by the lengths of. This video covers how to find the distance (r) and direction (theta) of the complex number on the complex plane, and how to use trigonometric functions and the pythagorean theorem to make the conversion. So for example, z = 6 + j4 represents a single point whose coordinates represent 6 on the horizontal real axis and 4 on the vertical imaginary axis as shown. Fly 45 miles ∠ 203 o (west by southwest). Web how to convert a complex number into rectangular form. All else is the work of man.” Drive 41 miles west, then turn and drive 18 miles south. What is a complex number? Your comments indicate that you're used to writing vectors, or points on a plane, with coordinates like ( a, b). This means that these are complex numbers of the form z = a + b i, where a is the real part, and b i represents the imaginary part.
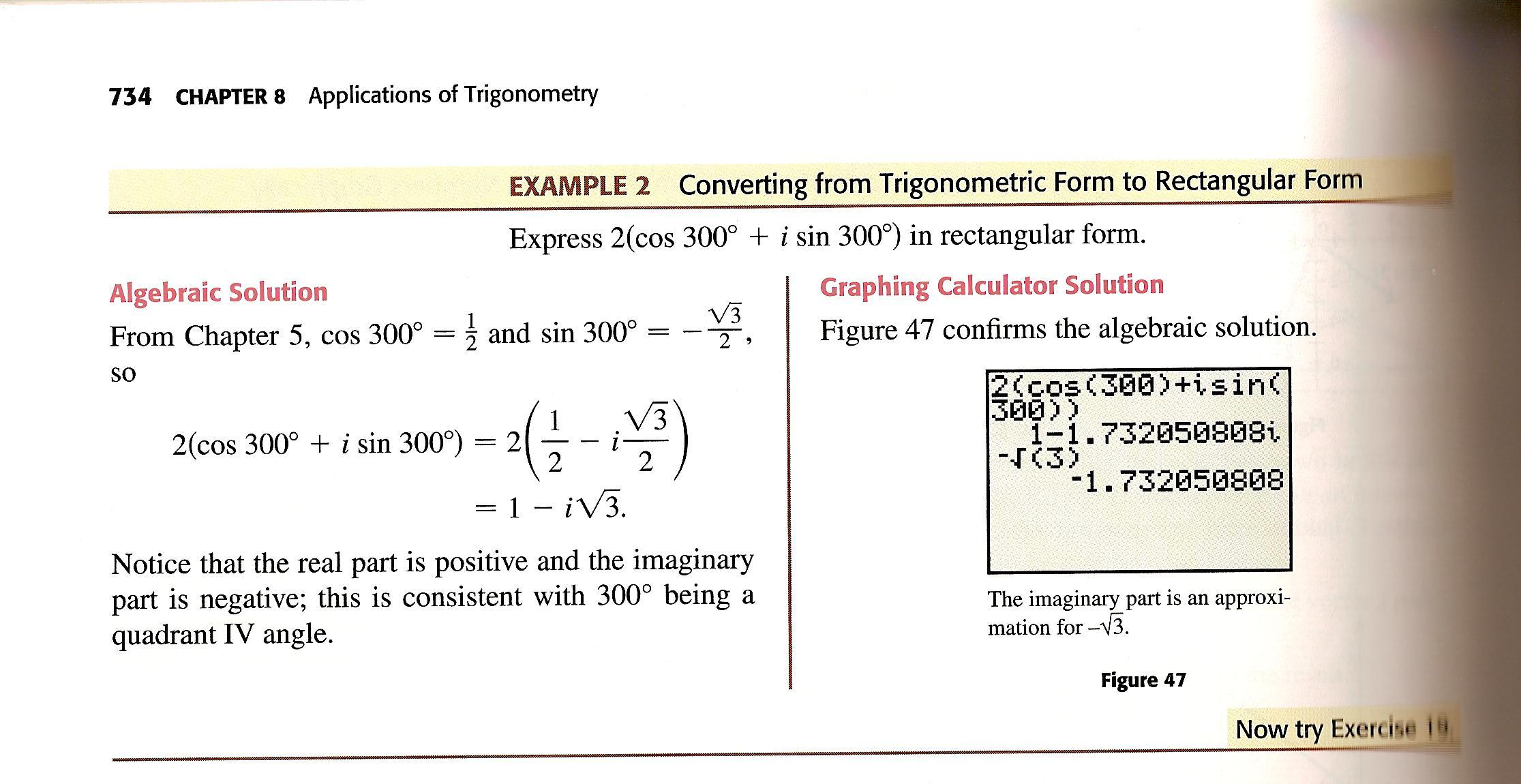
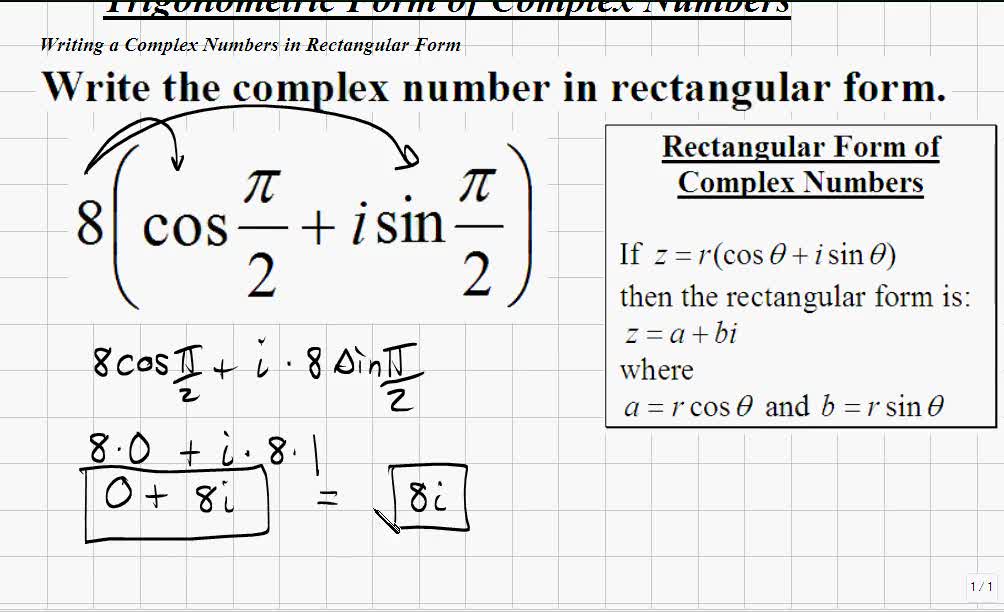
Solved Write the complex number in rectangular form. 8(cos
Drive 41 miles west, then turn and drive 18 miles south. This video covers how to find the distance (r) and direction (theta) of the complex number on the complex plane, and how to use trigonometric functions and the pythagorean theorem to make the conversion. Find products of complex numbers in polar form. In essence, the angled vector is taken.
Rectangular form vs. Trig/Polar form of a Complex Number TI 84
Web convert a complex number from polar to rectangular form. Fly 45 miles ∠ 203 o (west by southwest). Find products of complex numbers in polar form. There's also a graph which shows you the meaning of what you've found. If this were a point in the complex plane, what would be the rectangular and exponential forms of the complex.
2.5 Polar Form and Rectangular Form Notation for Complex Numbers
Z = x+iy (1.3.1) (1.3.1) z = x + i y 🔗 is called the rectangular form, to refer to rectangular coordinates. Web what is rectangular form? Web when dividing two complex numbers in rectangular form we multiply the numerator and denominator by the complex conjugate of the denominator, because this effectively turns the denominator into a real number and.
Rectangular Form Of A Complex Number Depp My Fav
This video covers how to find the distance (r) and direction (theta) of the complex number on the complex plane, and how to use trigonometric functions and the pythagorean theorem to make the conversion. Rectangular form for the complex numbers z1 = 3 4i and z2 = 7+2i, compute: Fly 45 miles ∠ 203° (west by southwest). #3*cos(120^@)+3*isin(120^@)# recall the.
Converting Complex Numbers from Rectangular to Polar Form YouTube
Z = r(cos(θ) + i ⋅ sin(θ)) we find that the value of r = 4 and the value of θ = π 4. (a) z1 z2 (b) z1 z2 (c) z1 z2 2 circle trig complex find the rectangular coordinates of the point where the angle 5ˇ 3 meets the unit circle. Find quotients of complex numbers in polar.
Complex Numbers (Rectangular & Polar) Operations YouTube
All else is the work of man.” Fly 45 miles ∠ 203 o (west by southwest). What is a complex number? Drive 41 miles west, then turn and drive 18 miles south. Here are some examples of complex numbers in rectangular form.
Rectangular Form Of A Complex Number Depp My Fav
Web this can be summarized as follows: Find products of complex numbers in polar form. The polar form of a complex number z = a + b i is z = r ( cos θ + i sin θ) , where r = | z | = a 2 + b 2 , a = r cos θ and b.
Complex numbers in rectangular form YouTube
The real part is x, and its imaginary part is y. Rectangular notation denotes a complex number in terms of its horizontal and vertical dimensions. Kelley's math & stats help. Web what is rectangular form? For background information on what's going on, and more explanation, see the previous pages, complex numbers and polar form of a complex.
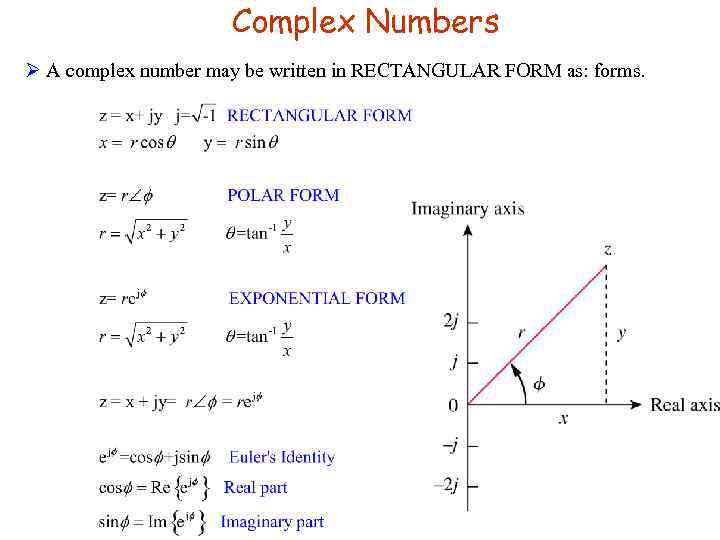
Complex Numbers and Phasors Chapter Objectives Ø Understand
All else is the work of man.” Web what is rectangular form? For example, 2 + 3i is a complex number. Which of these represents the same number in polar form? The number's \blued {\text {real}} real part and the number's \greend {\text {imaginary}} imaginary part multiplied by i i.
Complex Numbers Rectangular form YouTube
Polar notation denotes a complex number in terms of its vector’s length and angular direction from the starting point. As such, it is really useful for. Complex number polar form review. So for example, z = 6 + j4 represents a single point whose coordinates represent 6 on the horizontal real axis and 4 on the vertical imaginary axis as.
The Polar Form Of A Complex Number Z = A + B I Is Z = R ( Cos Θ + I Sin Θ) , Where R = | Z | = A 2 + B 2 , A = R Cos Θ And B = R Sin Θ , And Θ = Tan − 1 ( B A) For A > 0 And Θ = Tan − 1 ( B A) + Π Or Θ = Tan − 1 ( B A) + 180 ° For A < 0.
Web this can be summarized as follows: Polar notation denotes a complex number in terms of its vector’s length and angular direction from the starting point. Web when dividing two complex numbers in rectangular form we multiply the numerator and denominator by the complex conjugate of the denominator, because this effectively turns the denominator into a real number and the numerator becomes a multiplication of two complex numbers, which we can simplify. This means that these are complex numbers of the form z = a + b i, where a is the real part, and b i represents the imaginary part.
Find Powers Of Complex Numbers In Polar Form.
Kelley's math & stats help. Rectangular notation denotes a complex number in terms of its horizontal and vertical dimensions. 5\sqrt {2}\left ( \cos (135\degree) +i\sin (135\degree) \right) 5 2 (cos(135°) +isin(135°)) a 5\sqrt {2}\left ( \cos (135\degree) +i\sin (135\degree) \right) 5 2 Web convert a complex number from polar to rectangular form.
So For Example, Z = 6 + J4 Represents A Single Point Whose Coordinates Represent 6 On The Horizontal Real Axis And 4 On The Vertical Imaginary Axis As Shown.
#3*cos(120^@)+3*isin(120^@)# recall the unit circle coordinates: Find quotients of complex numbers in polar form. Web polar notation denotes a complex number in terms of its vector’s length and angular direction from the starting point. Your comments indicate that you're used to writing vectors, or points on a plane, with coordinates like ( a, b).
The Number's \Blued {\Text {Real}} Real Part And The Number's \Greend {\Text {Imaginary}} Imaginary Part Multiplied By I I.
Web to multiply two complex numbers z1 = a + bi and z2 = c + di, use the formula: There's also a graph which shows you the meaning of what you've found. Drive 41 miles west, then turn and drive 18 miles south. In essence, the angled vector is taken to be the hypotenuse of a right triangle, described by the lengths of.